Discrete vs. Continuous RVs (Random Variables)

• A discrete RV has a countable number of possible outcomes

  • number of students present

  • number of red marbles in a jar

  • number of heads when flipping three coins

  • students' grade level

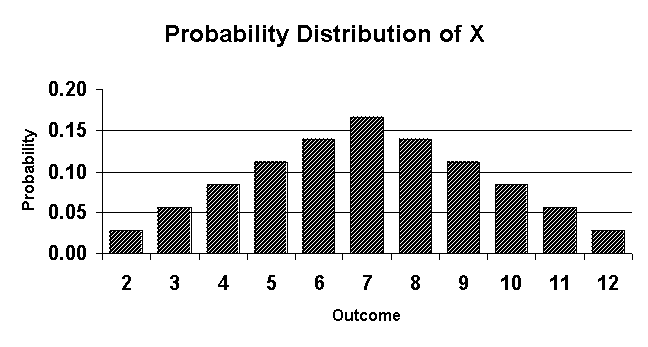
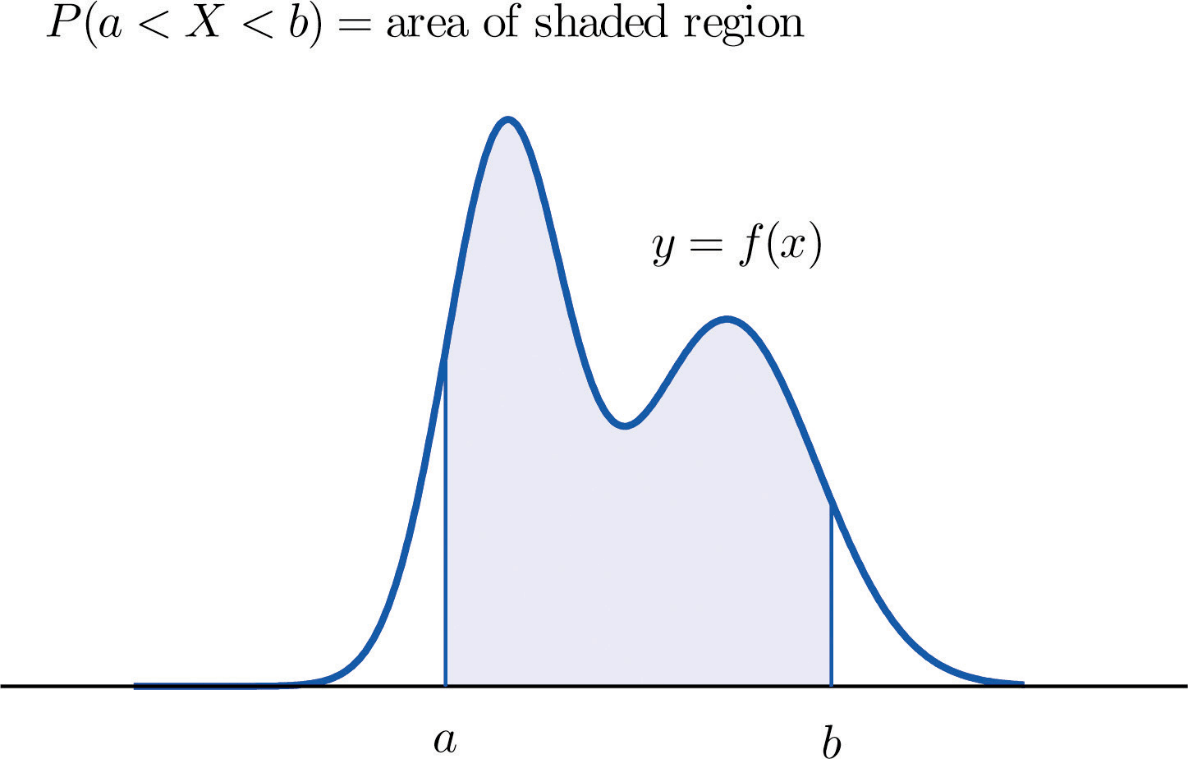
• A continuous RV can take any values in an interval of numbers

  • height of students in class

  • weight of students in class

  • time it takes to get to school

  • distance traveled between classes

  

Probability Distribution

• The probability distribution of a discrete RV(X) list all the values possible and their probabilities

  

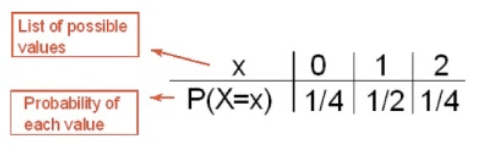
• The probabilities must:

  • All be a number between 0 and 1

  • Together add up to 1

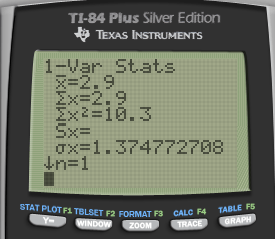
Mean = Expected Value

• Formula

  

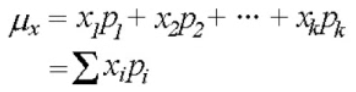
• Calculator

  • Type in X in L1 and P in L2

  

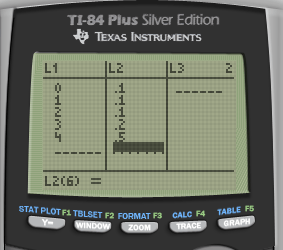
• 1-Var Stats L1, L2

  

Variance = Standard Deviation

• Formula

  

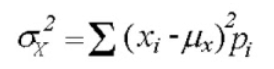
• Calculator

  

Practice Questions

• A bin contains ten $1 bills, five $2 bills, three $5 bills, one $10 bill, and one $100 bill. A person is charged $10 to select one bill. Let the random variable (X) be the amount someone wins by playing.

  • Construct a probability distribution for these data.

  

• What is the mean and standard deviation of the amount of money someone can expect to win?

  

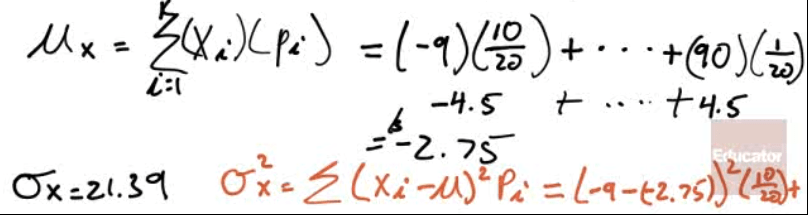
• You work for a company and are tasked with evaluating a proposed venture. The venture stands to make a profit of $10,000 with probability 4/20, to make a profit of $5200 with probability 8/20, to break even with probability 1/4, and to lose $5000 with probability 3/20. The expected profit in dollars is? Would you recommend this venture?

  

  

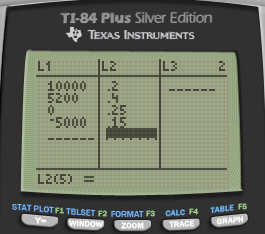
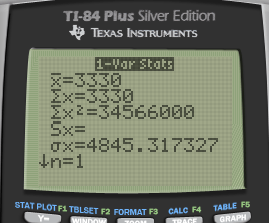
• Answer: The expected profit is $3330. Recommend, because the standard deviation is not so large